Next: Introduction
sweetser@alum.mit.edu
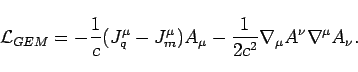
The GEM Lagrangian has mass and electric current densities coupled
to the 4-potential with different signs: like charges attract for
gravity and repel for electromagnetism. A four-dimensional wave equation
results by varying the action with respect to the potential:
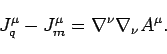
If the mass current density ![]() is zero, one sees the Maxwell
equations. If the electric charge density
is zero, one sees the Maxwell
equations. If the electric charge density ![]() is zero but
the mass density
is zero but
the mass density ![]() is not, in the static case, Newton's
field equation for gravity results. When a constant potential is chosen,
for a metric compatible, torsion-free connection, the exponential
metric tensor solves the field equations:
is not, in the static case, Newton's
field equation for gravity results. When a constant potential is chosen,
for a metric compatible, torsion-free connection, the exponential
metric tensor solves the field equations:
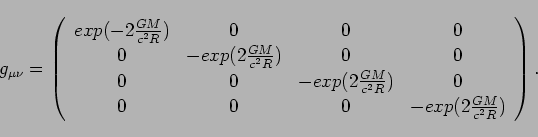
The exponential metric equation has the same ten parameterized post-Newtonian (PPN) coefficients as the Schwarzschild metric, so it will pass all the same tests of the equivalence principle and the metric. The second-order PPN coefficients for the two metrics differ. Light will bend around the Sun 0.7 microarcseconds more for this proposal than for the Schwarzschild metric, beyond our means to measure today. Energy loss by gravitational waves should be consistent with the proposal. When a flat metric is chosen, a normalized, linear perturbation potential function is found whose derivative has the correct inverse squared distance dependence needed for a classical force.
Analysis of the gravitational Lorentz force indicates both the standard mass times acceleration effect and a new classical gravitational effect, velocity times the change in passive inertial mass with respect to space. This may lead to new explanations for the rotation profile of thin galaxies without requiring dark matter or a modification of Newtonian mechanics (MOND).
Quantization will be very similar to the Gupta-Bleuler method of fixing the Lorenz gauge. The key difference is that the GEM field equations have two spin fields, one even, the other odd. The polarization of gravity waves are different than the transverse waves predicted by general relativity, setting the stage for a future test of the proposal.
12.10.-g