Douglas B Sweetser*
39 Drummer Road, Acton, MA 01720
version 20091002
Abstract
The Maxwell action of electromagnetism is rewritten using the noncommutative division algebra
of quaternions. The potential in the electromagnetic action is rewritten with the weak force
gauge symmetry SU(2), also known as the unit quaternions. The potential can be recast again
with electroweak symmetry as the product of U(1) and SU(2) symmetries. The conjugate of
one electroweak symmetry times another for the potential in the action is enough to account
for the strong force symmetry SU(3). A 4D commutative division algebra is constructed from
the hypercomplex numbers modulo eigenvalues equal to zero. The action is rewritten again with
the hypercomplex multiplication rules in a gauge invariant way. Like charges attract for the
hypercomplex action based on an analysis of spin of the field strength density, the spin in the phase
of the current coupling term, and the field equations that result by applying the Euler-Lagrange
equation. The field equation of the hypercomplex action contains Newton’s law of gravity paired
with a time-dependent term and thus consistent with special relativity. There is also an Ampere-like
equation so that a 4-potential theory can account for bending of both time and space caused by
gravity. It is shown how the Rosen metric is a solution to the field equations, and thus passes weak
field tests of gravity to first-order Parametrized Post-Newtonian (PPN) accuracy. The proposal
is distinguishable from general relativity at second-order PPN accuracy. The lowest mode of
wave emission for this simple field theory is a quadrupole. The final rewrite of the action has
gauge-dependent electromagnetic and gravity field strength densities where the two gauges cancel
out, leaving a gauge-independent unified action. Several issues that arise with quantization are
discussed.
_______
*Electronic address: sweetser@alum.mit.edu
Experiment tells us that the effects of gravity can be accurately described by a dynamic metric. A metric is symmetric, so changes in a dynamic metric are also symmetric. Quaternions will not suffice for the task. Frobenius proved long ago that the only finite, associative division algebras over the real numbers are the real numbers, the complex numbers, and the quaternions, up to an isomorphism. In these three cases, a set of zeros must be excluded to assure multiplication has an inverse. I hope to demonstrate that the hypercomplex numbers modulo eigenvalues equal to zero will always have an inverse. Such numbers form a 4D commuting division algebra that is not isomorphic to the quaternions. The hypercomplex rules of multiplication are used in the Maxwell action. The resulting action has like charges attract as happens for gravity. A metric solution is found which is consistent with weak field tests, yet different for higher order experimental tests. General relativity may have a new competitor, a symmetric variation of Maxwell.
In this paper, tensors are upgraded to have the power to be multiplied together. Such a goal is constrained for finite algebras to 1, 2, or 4 dimensions over the real numbers. Quaternions are a mathematical field, meaning they are a group under the addition operator, and modulo division by zero, a group under multiplication. In four dimensions, quaternions are isomorphic to tensors in that they can be added together, multiplied by a scalar and be written in an arbitrary choice of coordinate systems.
The standard way to write the Maxwell action without a source has the Lorentz invariant difference between the squares of two fields:
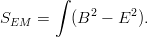 | (1) |
If a quaternion differential operator acts on a quaternion potential, one gets a gauge term
(g ≡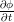 -∇A), an electric, and a magnetic field:
-∇A), an electric, and a magnetic field:
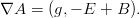 | (2) |
It is a simple exercise to make eq. 2 invariant under a gauge transformation by subtracting away the scalar, ∇A- (∇A)*. The sign of the magnetic field can be flipped by reversing the order of the differential and the potential. The product of the differential and the potential written in both orders will generate the difference of two squares. Another riddle is how to ensure U(1) symmetry for the current coupling term. As usually taught, U(1) symmetry is the unit circle in the complex plane. Quaternions can be considered 3 complex numbers that share the same real value. A normalized quaternion represents a unit circle of a complex plane at some angle in space. Use these observations to construct the Maxwell action using real-valued quaternions:
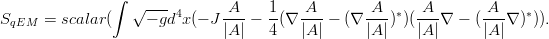 | (3) |
The 3-vector portion is the Poynting vector which provides a measure of the change in energy density in space caused by the electric and magnetic fields.
The gauge symmetry for the weak force is the non-Abelian group SU(2) also known as the unit quaternions. One way to represent the group is with the exponential of the 3-vector of a quaternion. Consider the following transformation of the electromagnetic action:
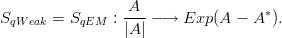 | (4) |
Both the current coupling and field strength density terms are guaranteed to have SU(2) symmetry. Where there is symmetry in the action, there are conserved currents. Since the Lie algebra su(2) has three generators, there are three conserved charges, the W+, W-, and Z.
The electroweak force sounds like the product of the electromagnetic and weak symmetries. Yet the first is an Abelian group while the second is not. Quaternions are generally non-Abelian. They are Abelian if two quaternions happen to point in the same direction, which always happens when there is only one quaternion involved. The electroweak symmetry in an action can be achieved with the following transformation of the electromagnetic action:
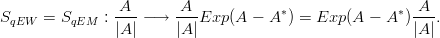 | (5) |
The normed quaternion which represents U(1) symmetry commutes with the exponential representation of SU(2) because both point in the same direction. Together, their Lie algebras exhaust the four degrees of freedom found in one quaternion potential.
The strong force has a Lie algebra su(3) with eight generators, which sounds like a pair of electroweak symmetries. Yet by group theory, multiplying one electroweak symmetry by another will generate a third member of the same group. One needs a new multiplication table, which can be done using a conjugate operator in the following way:
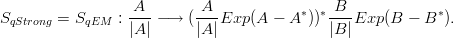 | (6) |
This has a norm of one and depends on eight independent elements in the two quaternion potentials A and B. Requiring the conjugate operator for multiplication makes this operation non-associative because (ab)*c≠a*(bc), although the norms are equal. Considerable effort has been directed towards finding a group larger than the standard model to include all the symmetries involved in the forces of Nature, from SU(5) to SO(10), even the group E8. [7] Only photons and the weak field particles have been isolated. There is no reason why confinement must exist for the strong force, which has hidden from view isolated quarks. If the only way to have the symmetry of the strong force is to necessarily have two 4-potentials, such a constraint might justify what has been observed. Much more work will need to be done to see if a smaller model can pass the success already achieved by the standard model.
One needs a division algebra that is more symmetric than quaternions. A division algebra is required because in quantum field theory, if one can invert the field equations, then one can find the propagator. Working with a 4D division algebra would assure the propagator exists. Since the antisymmetry in quaternion multiplication arises from differences in signs, construct an algebra where the signs of all products are positive. The familiar i2 equals +1, and ij = ji = 1. The hypercomplex numbers have these rules, although there are variations. [3] Because every value is positive, call this the California representation of hypercomplex numbers. A real 4x4 matrix representation is shown:
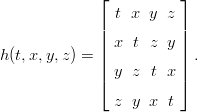 | (7) |
This matrix is one way to represent the Klein 4-group, the smallest non-cyclic group which is not a division algebra. Calculate the inverse of this matrix.
The divisor of the inverse is the product of the four eigenvalues of the matrix representation.
| Eigenvalue | Eigenvector |
| t + x + y + z | {1, 1, 1, 1} |
| t + x - y - z | {-1, -1, 1, 1} |
| t - x - y + z | {-1, 1, 1, -1} |
| t - x + y - z | {-1, 1, -1, 1} |
The hypercomplex numbers modulo the eigenvalues that equal zero will necessarily have an inverse. That is a defining property of a division algebra. The real number field mods out these same sets of numbers because the sum is zero which does not have an inverse. The graph for the California representation of hypercomplex numbers is a non-directional version of the quaternion graph as shown in figure 1. Quaternions are accepted as a mathematical field. The change to a hypercomplex field is that edges labeled with imaginary numbers become non-directional. The self-loops indicate these are not simple graphs. What should be simple is to establish hypercomplex analysis since the definition of a derivative will be the same as for real and complex analysis. The self-loops are essential since much of calculus involves infinitesimal change, or no change at all, which can be achieved with the self-loops.
This paper provides a counter-example to Frobenius’ theorem that the only associative, finite dimensional division algebras over the real numbers are the real numbers, complex numbers, and the quaternions, up to an isomorphism. A weakness in the proof may involve the assumption that imaginary numbers with i2 = -1 exist for the hypercomplex numbers, which is not the case according to its group multiplication table, eq. 7. There may be much for mathematicians to debate, but let us return to the physics.
Use the hypercomplex division algebra to form an action such that the scalar is Lorentz invariant. This can be achieved by using a conjugate operator. Addition and subtraction between 4-vectors is the same for quaternions as for hypercomplex numbers. What changes are the rules of multiplication. The difference in multiplication will be indicated by using a box times (⊠) for hypercomplex multiplication between 4-vectors, and an otimes (⊗) for the all-positive cross product between two 3-vectors.
 | (9) |
This action is invariant under a gauge transformation for exactly the same reason as eq. 3: the gauge terms are subtracted away for the field strength density, and the potential is normalized. The only difference between this action and the one for Maxwell is the conjugate operator acting on the potential and the change in the rules of multiplication. At first glance, one might think that such an action would result in like charges repelling. Reasons why like charges repel for electromagnetism will be compared with what happens for the hypercomplex action.
For the field strength density of electromagnetism, if the order of the differential operator is switched with the potential, then the sign of the B field changes. That indicates an odd spin particle mediates the force. The simplest such particle is the spin 1 photon. Switching the order of the differential operator and the potential under hypercomplex multiplication changes nothing, indicating an even spin, force-mediating particle. The field strength density is too complex to be spin 0, so a spin 2 particle is the simplest form for a mediating particle. Like charges attract for a spin 2 particle.
The second reason like charges repel for electromagnetism concerns the spin found by analyzing
the current coupling term, JA. One can take the Fourier transform of the 4-potential and write it
in the momentum representation as a current-current interaction, 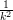 JJ′. Consider the phase of the
current-current term using quaternion and hypercomplex multiplication. For simplicity, focus on
the first component and omit the constant:
JJ′. Consider the phase of the
current-current term using quaternion and hypercomplex multiplication. For simplicity, focus on
the first component and omit the constant:
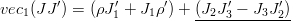 | (10) |
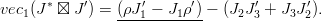 | (11) |
Both current-current interactions have two parts: one underlined once that works like a Lie algebra, being the difference of two densities, the other underlined twice a Jordan algebra, being the sum of two densities. The quaternion multiplication term underlined once should look familiar - it is angular momentum. The projection operator has a spin of 1. Note that because the action is invariant under a gauge transformation, there is no spin 0 state. This term is the relativistic correction for motion along the J1 direction. The terms with spin 1 symmetry for the charge density along the J1 direction arise oddly enough from the hypercomplex product, equation 11. The remaining terms have spin 2 symmetry. Because the terms add together constructively, they will travel through phase space twice as fast, needing only a 180 degree rotation to return. Like charges repel for forces mediated by spin 1 particles, and attract for spin 2.
The field equations that result from varying the action with respect to the potential are a third way to determine how like charges will respond to each other. In the static case for Gauss’ law, the charge density has a different sign from the Laplacian operator acting on the scalar potential, which means that like charges will repel. The field equations are generated for the hypercomplex action using the Euler-Lagrange equations:
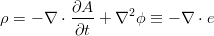 | (12) |
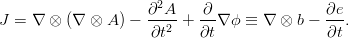 | (13) |
Because the charge density has the same sign as the Laplacian, like charges attract for the hypercomplex action. Two new fields have been defined, small e and small b which have the same differentials as their electromagnetic counterparts, just a few important sign changes. There are no corresponding vector identity equations for the hypercomplex action.
A flaw of Newton’s law of gravity is that it requires changes in the charge density to propagate instantaneously, which is not consistent with special relativity. One road to Einstein’s field equations is to make Newton’s law respect special relativity. [16] Because the hypercomplex field equations have time-dependent terms and is manifestly covariant, this motive for general relativity is eliminated.
There are articles in the literature that assert without proof that one cannot form vector field equations where like charges attract. [6] [13] Such work may assume using rules of algebra isomorphic to quaternions, and thus miss the results from the hypercomplex multiplication approach.
A gauge choice - how one measures things - for the hypercomplex action concerns how to measure a covariant derivative: how much should be due to the standard derivative versus how much is due to the connection, the changes in the metric? If one chooses to work with a flat Minkowski metric in Euclidean spacetime, the connection will be zero everywhere. For a static point charge, one gets both Newton’s law of gravity and three equations similar to Ampere’s law. The hypercomplex Ampere’s law are essential since gravity bends both time and space, while Newton’s law applied to a scalar field can only alter time. While it is impossible to formulate a scalar potential field theory consistent with light bending experiments, it is a simple task to do so with a 4-potential.
In the preceding paragraph, a choice was made that everything was due to the standard derivative of the potential. This time allow all of the change be due to the connection, nothing due to the standard derivative of the potential because the potential happens to be constant in time and space. Working with a constant potential transforms the hypercomplex field equations into the divergence of the connection:
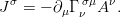 | (14) |
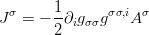 | (15) |
The metric must reduce to the Minkowski metric as the gravitational mass goes to zero. The exponential metric below provides a solution with these properties:
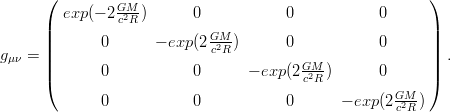 | (16) |
This is known as the Rosen metric, or the exponential metric. [12] The exponential metric is not a solution to the Einstein field equations, but has been studied in the literature because it is consistent with experimental tests of gravity to first order parametrized post-Newtonian (PPN) accuracy. [15] At second order PPN accuracy, eq. 16 predicts 0.7μmicroarcseconds more bending of star light by the Sun. [4] An experiment such as LATOR, could confirm or reject this proposal on experimental grounds. [14]
The bimetric theory of Rosen is not consistent with strong field tests of gravity. The fixed background metric can store momentum, allowing for dipole modes of emission of gravity waves which are ruled out by observations of binary pulsars. [17] The hypercomplex action does not have an additional field to store momentum. For an isolated source, the lowest mode of emission that conserves momentum will be the quadrupole mode which is consistent with observation.
Find a solution to both the Maxwell and hypercomplex field equations. There are two gauge choices that need to be made. The first gauge choice has to do with the diffeomorphism symmetry: how much change is in the potential versus the connection? For simplicity, let us work with all the change in the potential. The diffeomorphism symmetry in the action is the reason mass is conserved. The second gauge symmetry has to do with the potential, and is the reason electric charge is conserved. Choose to work in the Lorenz gauge. The Maxwell equations become a simple 4D wave equation. The hypercomplex field equations in this gauge have all the same terms:
If the hypercomplex equations are subtracted from the Maxwell equations, then in each
equation, one term cancels out of the form  , the diagonal term. A 4-potential solution can be
found:
, the diagonal term. A 4-potential solution can be
found:
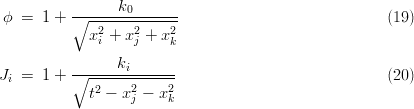
The scalar potential solution is perhaps the best known in physics, often the first one taught when learning the subject. The constant 1 is needed so the energy density of the field is positive whether k is positive as must be for like charges to repel in electromagnetism, or k is negative when like charges attract in gravity, an issue appreciated by Maxwell. [2] One needs the three spatial derivatives to magically cancel out with the second derivatives. The trick needs a slight modification due to the differences in sign between the time and space elements, but again three variables are needed for the solution. By excluding the one derivative that is different between the Maxwell and hypercomplex field equations, a postive energy potential that solves both field equations is found.
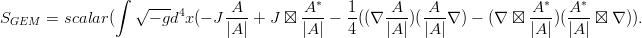 | (21) |
Both field strength densities generate the same scalar that is the square of a gauge,
(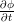 -∇⋅ A)2. The difference of the two actions makes a unified action that is invariant under a
gauge transformation. Gauge symmetry is broken separately for electromagnetism and gravity,
while being maintained for the unified GEM action. This is accomplished without the Higgs
mechanism. The GEM proposal predicts the Higgs boson will not be found in particle field
experiments such as the Large Hadron Collider (LHC).
-∇⋅ A)2. The difference of the two actions makes a unified action that is invariant under a
gauge transformation. Gauge symmetry is broken separately for electromagnetism and gravity,
while being maintained for the unified GEM action. This is accomplished without the Higgs
mechanism. The GEM proposal predicts the Higgs boson will not be found in particle field
experiments such as the Large Hadron Collider (LHC).
The field equations are simplified due to the subtraction:
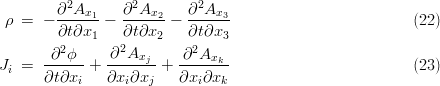
As done earlier, if one works in the gauge for the covariant derivative where all change is in the potential not the metric, and the Lorenz gauge for the 4-potential, then one gets the following field equations:
The unified GEM field equations may appear too simple. Yet the field equations strive to be a structure general enough to account for any force in Nature, no matter how that force works. Forces in turn involve second order differential equations. The action that leads to these field equations can have all the symmetries found in the standard model. The Maxwell equations on the other hand are appropriate for waves that travel at the speed of light. Particles with mass will need equations that reduce to Newton’s force equations in a classical limit. Simplicity is required to be flexible enough to cover all the ways the four known forces work.
The deep insight into gravity provided by general relativity is that the geometry of spacetime is not presumed. In special relativity, the metric must be constant, the same for all inertial observers. In general relativity, the metric can vary depending on where the observer is in spacetime: being closer to a mass source will have more spacetime curvature. The Riemann curvature tensor is a rank 4 tensor that contains second order derivatives of the metric. The Hilbert action has the Ricci scalar, a contraction of the Riemann curvature tensor. By varying the Hilbert action with respect to the metric tensor, the geometry of spacetime can change as dictated by the Einstein field equations.
Electromagnetism works no matter what the metric happens to be. The antisymmetric tensor cannot characterize how the symmetric metric tensor changes, so the possibly dynamic metric must be supplied as part of the background mathematical structure. By using hypercomplex multiplication for the GEM action, it becomes possible for the action to account for the changes in the metric tensor, and thus remove the metric from the background structure. The idea is not to treat the metric as an active field, but instead to use the diffeomorphism symmetry of the action to provide a constraint on a metric that varies in spacetime. One can choose how much of a covariant derivative is due to a dynamic potential or a dynamic metric. Where there is a symmetry, there is a conserved charge. Since the symmetry involves the metric, the charge must be mass. Energy and momentum also arise from symmetries in the action, the ability to vary the action in terms of time and location respectively. Since mass is the square of the energy minus the square of the momentum, it is logically consistent that energy, momentum, and mass all can be viewed as different symmetries of the same action.
Repeat the thought experiment, but this time make all the particles positively charged. Now the thought experiment cannot be done because it would involve destroying an electric charge. There are solid theoretical and experimental tests demonstrating that electric charge cannot be destroyed. [9] Because the thought experiment cannot be done, the conclusion is not supported.
The Maxwell equations in the Lorenz gauge is a 4D wave equation that has been quantized. [1, 5] Two spin 1 modes of emission must be made virtual to avoid a non-physical negative probability density. The gravity field equations proposed in this paper (eq. 17 and 18) have the same terms, with only one sign different. It may turn out that the virtual spin 1 field is actually a real spin 2 field for gravitons. The main difference would be the change from a spin 1 to a spin 2 propagator. More work will be required to see if this speculation is reasonable and well behaved.
The linear unified GEM field equations (eq. 24 and 25) are about as simple as they come. Any harmonic function could be a solution. It is straight forward to calculate the Hamiltonian ((B + b)(B - b)) and field strength tensor from the unified GEM action. This author is reluctant to take the next steps for quantization. One proof of Frobenius’ theorem on finite division algebras uses the fundamental theorem of algebra, a name for a theorem that does not invite alternatives. Yet hypercomplex numbers are designed specifically so there is no such product as i2 = -1 which plays a major part in the fundamental theorem of algebra. In quantum mechanics, eigenvalues are observables. It is an objective of calculations to determine how frequently those states are populated given the physical conditions. With hypercomplex numbers, it is unclear if the eigenvalues can ever be occupied since such states do not have inverses and would not be reversible. The use of two different rules of multiplication will present a challenge to establishing a mathematically rigorous quantum field theory.
There is a well-known thought experiment imagining a man in a closed box who would be unable to tell if he was in a smoothly accelerating rocket ship or the box was sitting on the surface of a planet (ignoring tidal effects). For the box on the planet, general relativity would be able to generate a metric equation that would, in a limit process, become Newton’s potential theory. For the GEM approach, one could choose a dynamic metric which would be equivalent to first order PPN accuracy to the metric from general relativity, or with the practical power of diffeomorphisms, choose to work in a flat spacetime and have a 4-potential completely characterize the gravitational field (see figure 2). A scalar potential like Newton’s law of gravity is inadequate because there is only one potential, so it gets the answer to light bending around the Sun half right. A 4-potential can describe the way measurements of both time and space are bent by a 4-momentum density.
The unified GEM action may strike some as being far too simple to account for all four known forces. Yet the simplest components in Nature adhere strictly to the rules. Particles cannot be programmed to do the right thing. Instead, the rules of accounting dictate what event can happen in spacetime. There is only one way to add 4-vectors together. With multiplication, there are more choices. Nature keeps multiple books so gravity, electromagnetism, the weak and strong forces, can all be done by the same simple particles. The action is the product of an operator and a 4-potential. In quantum field theory, an operator is an observable, the operator acting on the potential may have more freedom that many give it credit. Figuring out how to do 4D math correctly by trying to read Nature’s cards may yield more insights into how physics works at the most fundamental level.
The GEM action is to a surprising degree exactly what Einstein searched for over the last half
of his life. [10] He tried an impressive range of ideas, from five dimensional Kaluza-Klein
variations, to asymmetric Riemann curvature tensors, all to no avail. The radical approach used
here is to abandon the Riemann curvature tensor, and work only with the building blocks of the
curvature tensor, the connection, found in a covariant derivative. Efficient elegance governs the
heavens.
_______________________________________________
[1] K. Bleuler. Helv. Phys. Acta, 23:567, 1950.
[2] K. Brown. Why maxwell couldn’t explain gravity, 2000.
[3] C. M. Davenport. Clifford Algebras with Numeric and Symbolic Computations. Birkhauser,
1996.
[4] R. Epstein and I. I. Shapiro. Post-post-newtonian deflection of light by the sun. Phys. Rev.
D, 22(12):2947–2949, 1980.
[5] S. N. Gupta. Theory of longitudinal photons in quantum electrodynamics. Proc. Phys. Soc.,
63:681–691, 1950.
[6] S. N. Gupta. Einstein’s and other theories of gravitation. Rev. Mod. Phys., 29:337–350,
1957.
[7] A. G. Lisi. An exceptionally simple theory of everything. 2007.
[8] C. W. Misner, K. S. Thorne, and J. A. Wheeler. Gravitation. W. H. Freeman and Company,
1970.
[9] L. B. Okun. Tests of electric charge conservation and the pauli principle. Sov. Phys. Usp.,
32:543–547, 1989.
[10] A. Pais. ’Subtle is the Lord...’: The science and the life of Albert Einstein. Clarendon Press,
1982.
[11] R. H. Price. General relativity primer. 50(3):300–367, 1982.
[12] N. Rosen. A bi-metric theory of gravitation. General Relativity Gravitation, 4(6):435–447,
1973.
[13] W. E. Thirring. An alternative approach to the theory of gravitation. Ann. Phys. (U. S.
A.), 16:96–117, 1961.
[14] S. G. Turyshev, M. Shao, and K. L. Nordtvedt. Science, technology and mission design for
the laser astrometric test of relativity, 2006.
[15] Keith Watt and Charles W. Misner. Relativistic scalar gravity: A laboratory for numerical
relativity. 1999.
[16] S. Weinberg. Derivation of gauge invariance and the equivalence principle from lorentz
invariance of the s-matrix. Phys. Let., (9):357–359, 1964.
[17] C. M. Will. Theory and experiment in gravitational physics: Revised edition. Cambridge
University Press, 1993.
[18] C. M. Will. The confrontation between general relativity and experiment. Living Rev.s in
Relativity, 9(3), 2006.
Figure 1: Hypercomplex and quaternion graphs
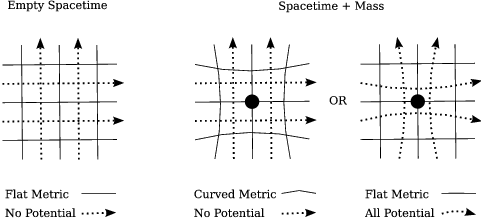
Figure 2: Curved metrics and/or 4-potentials for gravity